Differential forms and separated variables

Differential equations: Separation of variables

Differential forms and separated variables

We have seen that finding an antiderivative of the function $f(t)$ can be formulated as solving the first order differential equation $y'=f(t)$ . An effective intermediate step from the ODE to the solution turned out to be its differential form: $\dd y = f(t)\,\dd t$ . There are more first-order ODEs that can be solved using differential forms.

Separation of variables

Suppose that $f(t)$ and $g(y)$ are continuous functions, that $g(y)$ is not the constant function $0$ , that $F(t)$ is an anti-derivative of $f(t)$ , and that $H(y)$ an anti-derivative of $\frac{1}{g(y)}$ .

The general solution $y$ of the differential equation $y'(t)={g(y)}\cdot {f(t)}$ satisfies the equality $H(y)=F(t)+C$ where $C$ is a constant.

The differential equation $\frac{\dd y}{\dd t} = {g(y)}\cdot{f(t)}$ can be written in differential form as $\dfrac{1}{ g(y)}\,\dd y = f(t)\,\dd t$ and so it is equivalent to $\dd\bigl(H(y)\bigr) = \dd\bigl(F(t)\bigr)$ Consequently, $H(y)=F(t)+C$ for a constant $C$ .

If $g(y)$ is the constant function $0$ , then the left hand side of the ODE is equal to $0$ , so $y$ is a constant function. This case is easy to handle.

In general, this is not a solution of the ODE in the explicit form $y(t)=\text{a function of }t$ , but a relationship between the variables $y$ and $t$ . Sometimes you can derive an explicit solution from this relationship.

Separable differential equation

Let $y$ be a differentiable function of $t$ . A separable differential equation is a first order differential equation of first degree in which the derivative $y'$ can be factored as a product of a function $g(y)$ of the unknown dependent variable $y$ and a function $f(t)$ of the independent variable $t$ : $y'(t) =g(y) \cdot f(t)$

A solution such as $H(y)=F(t)+C$ of the above theorem is often referred to as an implicit solution of the ODE.

If there are initial conditions, the implicit solution can be used for finding corresponding values of $C$ .

Each autonomous ODE is separable. After all, such an ODE can be written as $y '(t) = g(y) \cdot f(t)$ with $f(t)$ equal to the constant function $1$ .

Below are some examples. Later we will discuss the method in greater detail and provide more examples.

Determine a function $y$ of $t$ having value $1$ at $0$ that satisfies the ODE $y' =\dfrac{1}{3}$

$y= \dfrac{1}{3}\; t + 1$

The differential equation is separable since, putting $g(y) = \frac{1}{3}$ and $f(t) = 1$ , we can rewrite it as
$3\; \dd y = 1\; \dd t$
After computing antiderivatives we have
$3 y = t + C$
Multiplying both sides by $\frac{1}{3}$ , we find
$y = \dfrac{1}{3}\; t + C$
This is the general solution of the differential equation.

This solution denotes a class of functions, all of which have a rate of growth one third. Geometrically, we can represent the class of functions with the following slope field.

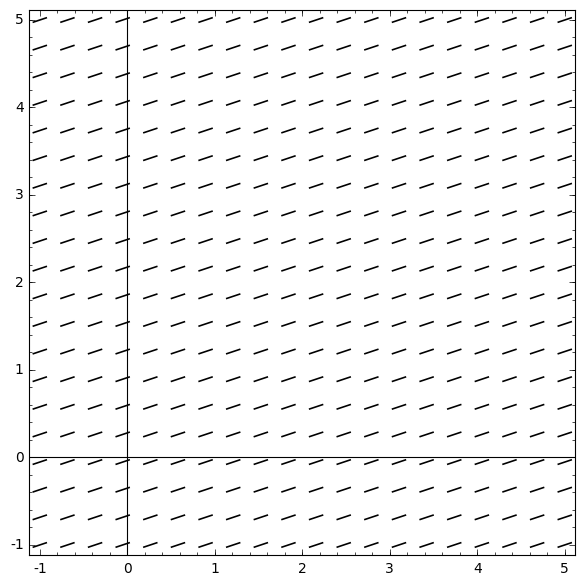
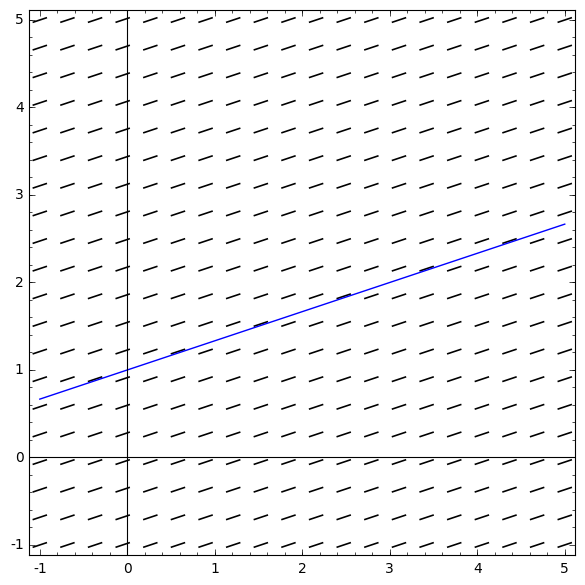
To obtain a specific solution we proceed to solve initial value problem with the initial condition $\rv{t,y} = \rv{0,1}$ . After substituting the values $y=1$ and $t=0$ we find
$1 = \dfrac{1}{3}\; \cdot 0 + C$
Therefore we have $C = 1$ , so the corresponding specific solution is the function:
$y = \dfrac{1}{3}\; t + 1$
This is represented graphically as a line inside the slope field.

New example