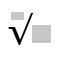Applications: Differentiation
Differentiation
Susan throws a basketball off a cliff that is #\unit{98}{m}# high. The height of the ball #h(t)# is measured in metres and the time #t# is measured in seconds. The ball leaves Susan's hand at #t =\unit{0}{s}#.
The height of the ball is given by the following function and graph below:
\[ h(t) = -4.9t^2 + 6 t + 98 \] You can click the throw button in the graph to see a visualisation of this.
The height of the ball is given by the following function and graph below:
\[ h(t) = -4.9t^2 + 6 t + 98 \] You can click the throw button in the graph to see a visualisation of this.
When the ball is moving upwards, it has a positive vertical velocity (speed). When the ball is moving downwards, it has a negative vertical velocity.
What is the vertical velocity of the ball just after it leaves Susan's hand?
Give your answer as an integer.
| velocity #=# | #\unit{}{m/s}# |
Unlock full access
Teacher access
Request a demo account. We will help you get started with our digital learning environment.
Student access
Is your university not a partner?
Get access to our courses via Pass Your Math independent of your university. See pricing and more.
Or visit omptest.org if jou are taking an OMPT exam.
Or visit omptest.org if jou are taking an OMPT exam.
