Intersection points of a parabola with a line

Quadratic equations: Intersection points of parabolas

Intersection points of a parabola with a line

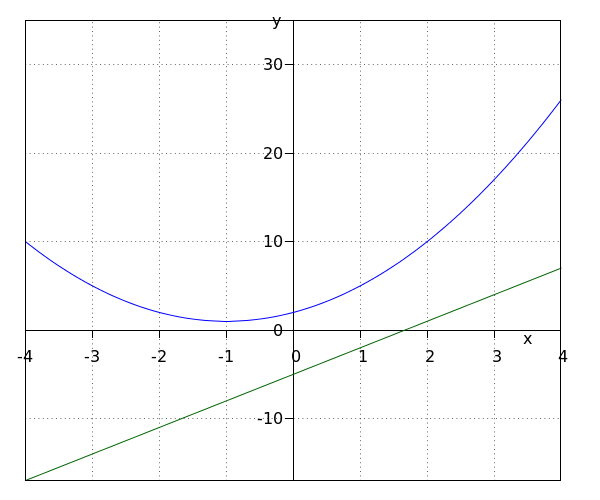
A quadratic formula $y=a_1x^2+b_1x+c_1$ and a linear formula $y=a_2x+b_2$ can have zero, one or two intersection points. We will now investigate how to find these intersection points.

Intersection points parabola and line

	Procedure	geogebra plaatje
	We determine the intersection point of the parabola $y=a_1x^2+b_1x+c_1$ and the line $y=a_2x+b_2$ .
Step 1	First, we determine the $x$ -coordinate of the intersection point by solving the equation $a_1x^2+b_1x+c_1=a_2x+b_2$ by means of factorization, completing the square or the quadratic formula.
Step 2	We determine the $y$ -coordinate of the intersection point by substituting the obtained $x$ -coordinate in one of both formulas. Usually, it is easier to substitute in the linear formula.

Number of intersection points and graphWe will now investigate how to recognize the number of intersection points between a parabola and a line. In step 1, we can calculate how many intersection points exist with the quadratic formula. Also, the number of intersection points is visible in the graph.

Formulas $y=x^2+2x+2$ $y=3x-5$	Formulas $y=x^2+2x+2$ $y=3x+\tfrac{7}{4}$	Formulas $y=x^2+2x+2$ $y=3x+4$
Corresponding equation $x^2+2x+2=3x-5$	Corresponding equation $x^2+2x+2=3x+\tfrac{7}{4}$	Corresponding equation $x^2+2x+2=3x+4$
Reduce to $0$ $x^2\green{-}x+\purple{7}=0$	Reduce to $0$ $x^2\green{-}x+\purple{\tfrac{1}{4}}=0$	Reduce to $0$ $x^2\green{-}x\purple{-2}=0$
Discriminant $\begin{array}{rcl}\orange D&=&\left(\green{-1}\right)^2-4 \cdot \blue1 \cdot \purple{7}\\&=&\orange{-27}\lt 0\end{array}$	Discriminant $\begin{array}{rcl}\orange D&=&\left(\green{-1}\right)^2-4 \cdot \blue1 \cdot \purple{\frac{1}{4}}\\&=&\orange{0}\end{array}$	Discriminant $\begin{array}{rcl}\orange D&=&\left(\green{-1}\right)^2-4 \cdot \blue1 \cdot \purple{-2}\\&=&\orange{9}\gt 0\end{array}$

No intersection points.	One intersection point (point of tangency): $\rv{\frac{1}{2}, \frac{13}{4}}$ .	Two intersection points: $\rv{-1,1}$ and $\rv{2,10}$ .

The last step indicates that given the graphs $y = x^2-10\cdot x+40\phantom{xxx}\text{ and }\phantom{xxx} y = 17\cdot x-142$
intersect each other at two points. Determine the two intersection points.

Give your answer in the form $\left\{\rv{a,b},\rv{c,d}\right\}$ , in which $a$ , $b$ , $c$ , $d$ are exact numbers.

$\left \{\rv{ 13 , 79 } , \rv{ 14 , 96 } \right \}$

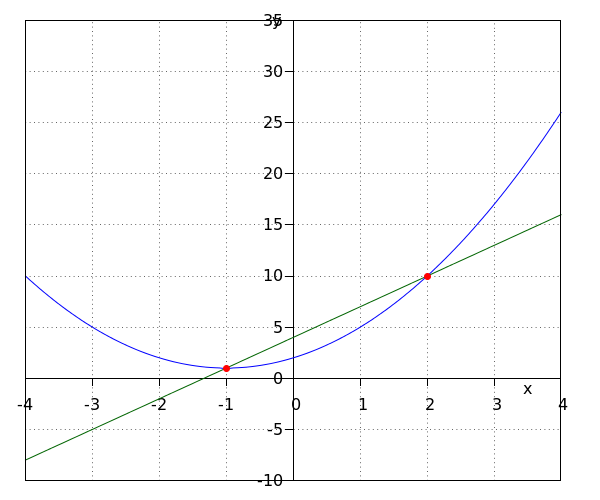
The $x$ -coordinate of a point lying on both parabolas must satisfy
$x^2-10\cdot x+40 = 17\cdot x-142\tiny.$
We solve this equation, after reduction, by factorization.
$\begin{array}{rcl} x^2-27\cdot x+182 &=& 0\\ &&\phantom{xxx}\blue{\text{all terms to the left hand side}}\\ \left(x-14\right)\cdot \left(x-13\right) &=&0 \\ &&\phantom{xxx}\blue{\text{factorized}}\\ x-14 = 0 &\lor& x-13=0 \\ &&\phantom{xxx}\blue{A\cdot B=0 \text{ if and only if }A=0\lor B=0}\\ x=14 &\lor& x=13 \\ &&\phantom{xxx}\blue{\text{constant term to the right hand side}}\\ \end{array}$
Now, we can calculate the corresponding $y$ -value by entering this $x$ -value in one of both formulas. In this case, it is most convenient to choose the linear function. First, we calculate the $y$ -value at $x=13$ .
$\begin{array}{rcl} y&= & 17\cdot 13-142 = 79 \end{array}$
Next, we calculate the $y$ -value at $x=14$ .
$\begin{array}{rcl} y&=& 17\cdot 14-142 = 96 \end{array}$
The conclusion is that the $2$ points of intersection are given by: $\left \{\rv{ 13 , 79 } , \rv{ 14 , 96 } \right \}\tiny.$

We see that the calculated intersection points match the intersection points identified in step 1. See the figure below, in which the intersection points are drawn in red.

New example