Inverse functions

Functions: Power functions and root functions

Inverse functions

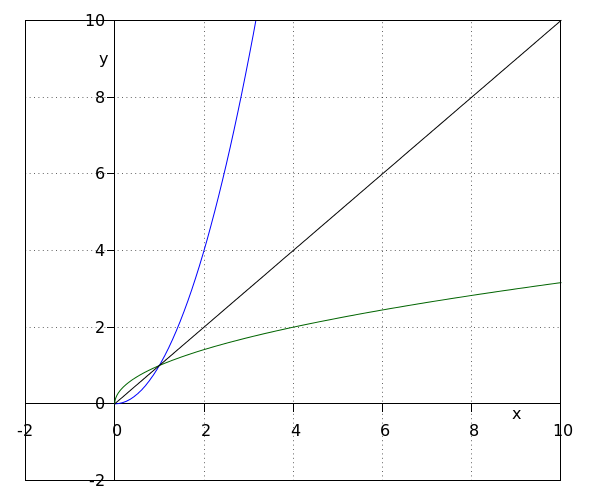
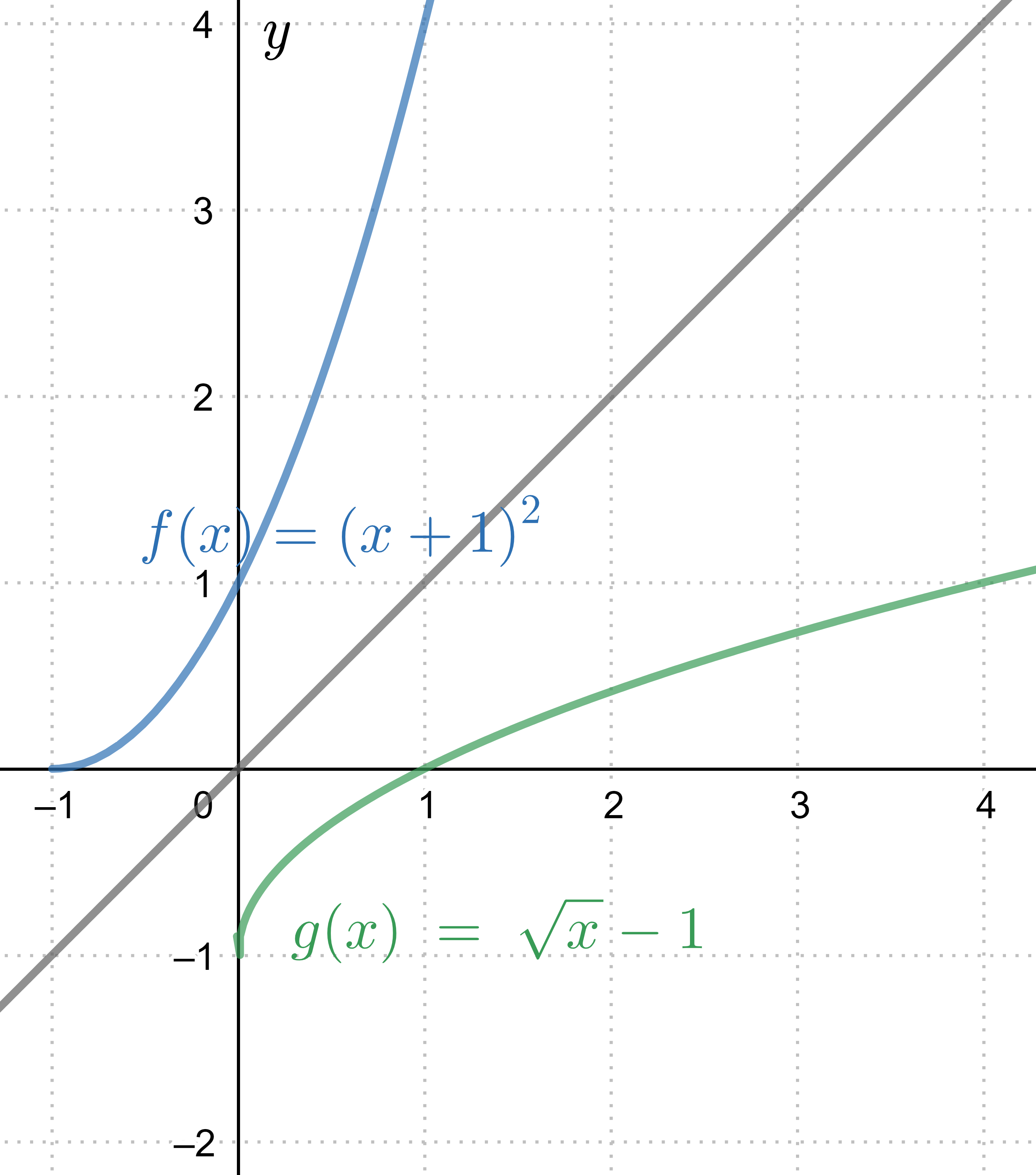We take a look at the functions $\blue{f(x)}=\blue{x^2}$ (solid) and $\green{g(x)}=\green{\sqrt{x}}$ (dashed) on the domain $\ivco{0}{\infty}$ .

For these functions we have $\blue f(\green{g(x)})=\left(\green{\sqrt{x}}\right)^\blue2=x$ for all $x$ in $\ivco{0}{\infty}$ .

Therefore $\green{g(x)}$ is called the inv‍erse of $\blue{f(x)}$ .

We also have $\green g(\blue{f(x)})=\green{\sqrt{\blue{x^2}}}=x$ for all $x$ in $\ivco{0}{\infty}$ .

Therefore $\blue{f(x)}$ is called the in‍verse of $\green{g(x)}$ .

In‍verse

The function $\blue{f(x)}$ has inv‍erse function $\green{g(x)}$ if $\blue f(\green{g(x)})=x$

From a geometrical perspective, the graph of the inverse of $\green{g(x)}$ is the reflection of $\blue{f(x)}$ over the line $y=x$ .

We can also notate the inverse of $f(x)$ by $f^{-1}(x)$ .

When determining the inverse of a function, the domain of that function is important. The domain of $\blue{f(x)}$ is the range of $\green{g(x)}$ and the domain of $\green{g(x)}$ is the range of $\blue{f(x)}$ . Hence, the inverse function $\green{g(x)}$ is defined on the range of function $\blue{f(x)}$ .

Example
In the graph $\blue{f(x)}=\blue{(x+1)^2}$ on the domain $x \ge -1$ and its inverse $\green{g(x)}=\green{\sqrt{x}-1}$ on the domain $x \ge 0$

Domain

The domain of the function $\blue{f(x)}=\blue{(x+1)^2}$ consists of all numbers and its range consists of all non-negative numbers. Its inverse function $\green{g(x)}=\green{\sqrt{x}-1}$ has thus as domain only the non-negative numbers, where its range is also only the non-negative numbers. Hence, the function $\blue{f(x)}=\blue{(x+1)^2}$ only has an inverse on the domain of all non-negative numbers.

The other way around we have that the function $\green{g(x)}=\green{\sqrt{x}-1}$ has function $\blue{f(x)}=\blue{(x+1)^2}$ as inverse, but only on the domain of all numbers greater than or equal to $-1$ . Because, that is the range of $\green{g(x)}$ and thus the domain of $\blue{f(x)}$ .

We will usually not mention these limitations in the domain of the inverse function, but implicitly assume them.

Determining inverse function

	Procedure We determine the inverse function $\green{f^{-1}(x)}$ of the function $\blue{f(x)}$ .	Example $\blue{f(x)}=\left(x-4\right)^2$ .
Step 1	Write the function as a formula, hence in the form $y=\ldots$ .	$y=\left(x-4\right)^2$
Step 2	Isolate the variable $x$ in the formula $y=\ldots$ . This means that the formula is written as $x=\ldots$ .	$x=\sqrt{y}+4$
Step 3	In the formula, change the $y$ into $x$ and $x$ into $y$ .	$y=\sqrt{x}+4$
Step 4	Replace $y$ by $\green{f^{-1}(x)}$ .	$\green{f^{-1}(x)}=\sqrt{x}+4$

Elaboration step 2 example

We work out step 2 from the example. In there we want to isolate the variable $x$ in the formula $y=\left(x-4\right)^2$ . This is done by means of reduction.

$\begin{array}{rcl}y&=&\left(x-4\right)^2 \\ &&\phantom{xxx}\blue{\text{original formula}} \\ \sqrt{y}&=&x-4 \\ &&\phantom{xxx}\blue{\text{both sides root extracted }} \\ \sqrt{y}+4&=&x \\ &&\phantom{xxx}\blue{\text{both sides plus }4}\end{array}$

Therefore, $x=\sqrt{y}+4$ .

Isolating a variable

Note that isolating a variable is actually the same as determining the inverse function.

In that case we don't have to go through step 3 and 4 anymore, and we are ready at step 2 of the procedure.

Extracting the root

In step 2, we took the square root on both sides to be able to isolate $x$ out of the square. We take the positive root. As we saw in the introduction of the root function the root of a non-negative number is a non-negative number.

If a certain domain is specified for finding the inverse, it could be necessary to take the negative root. In the example, $\blue{f(x)}$ has a domain of all real numbers and a range of all non-nagative numbers. The inverse function $\green{f^{-1}(x)}$ has as domain all non-negative numebrs and as range all number greater than or equal to $4$ . Thus, only on the domain $x \ge 4$ does $\blue{f(x)} = \blue{(x-4)^2}$ have the inverse $\green{f^{-1}(x)} = \green{\sqrt{x}+4}$ .
If we were asked to calculate the inverse of $\blue{f(x)}$ on the domain $x\le 4$ then we should have taken the negative root in step 2. The inverse of $\blue{f(x)} =\blue{ (x-4)^2}$ on the domain $x \le 4$ is $\green{f^{-1}(x) }= \green{-\sqrt{x}+4}$ . this function has as domain all non-negative numbers and as range all number less than or equal to $4$ , which is exactly the given domain.

Isolate $x$ in
$y=\sqrt{2\cdot x+1}$
Give your answer in the form $x = ...$

$x={{y^2-1}\over{2}}$

$\begin{array}{rcl} y&=&\sqrt{2\cdot x+1} \\ &&\phantom{xxx}\blue{\text{original function}}\\ y^2&=& 2\cdot x+1 \\ &&\phantom{xxx}\blue{\text{both sides squared}}\\ y^2-1&=&2\cdot x \\ &&\phantom{xxx}\blue{\text{both sides minus }1}\\ {{y^2-1}\over{2}} &=&x \\ &&\phantom{xxx}\blue{\text{both divided by }2}\\ x&=&{{y^2-1}\over{2}} \\ &&\phantom{xxx}\blue{\text{left and right swapped }}\\ \end{array}$

New example