Graph of logarithmic function

Exponential functions and logarithms: Logarithmic functions

Graph of logarithmic function

Previously, we look at the exponential function and its corresponding graph. We'll now study the graph of the logarithmic function. We'll see some interesting commonalities between this graph and the graph of the exponential function.

A function of the form $f(x)=\log_\blue{a}\left(x\right)$ with $\blue{a}>0$ , is called a logarithmic function.

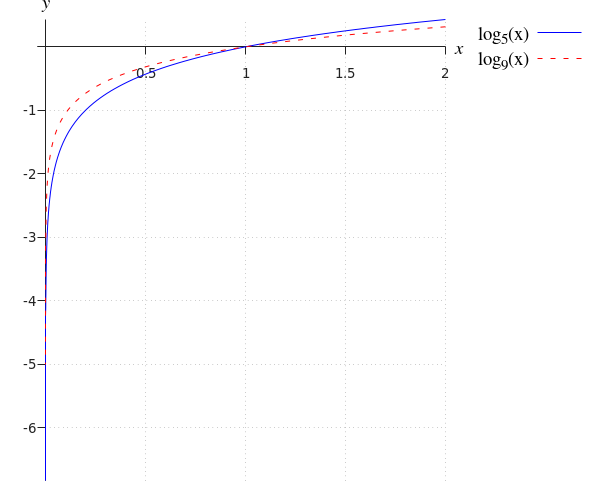
The graph's asymptote is the $y$ -axis. The logarithm is not defined for $x=0$ , but it is defined for positive numbers very close to zero.

Dependent on the value of $\blue{a}$ , the graph is either increasing or decreasing. If $\blue{a}>1$ , the graph is increasing. If $0<\blue{a}<1$ , the graph is decreasing.

We note that the domain of the logarithmic function is only the positive $x$ -axis, and its range the entire $y$ -axis.

geogebra plaatje

Draw the intersection point of the graphs of the functions $\log_{5}(x)$ and $\log_{9}(x)$ .

$\rv{1,0}$

The given values of the base numbers are irrelevant: the graph of $\log_a(x)$ will always move through the point $\rv{1,0}$ , as long as $a$ is positive, because $a^0=1$ .

New example