Transformations of power functions with negative exponents

Functions: Fractional functions

Transformations of power functions with negative exponents

We have seen the shape of the graph of a power function $f(x)=\tfrac{1}{x^n}$ with $n \gt 0$ and $n$ is an integer. Just as power functions of the form $y=x^n$ with $n \gt 0$ and $n$ is an integer, we can transform these power functions.

We can transform the function $f(x)=\frac{1}{x^n}$ in three different ways.

	Transformations	Examples
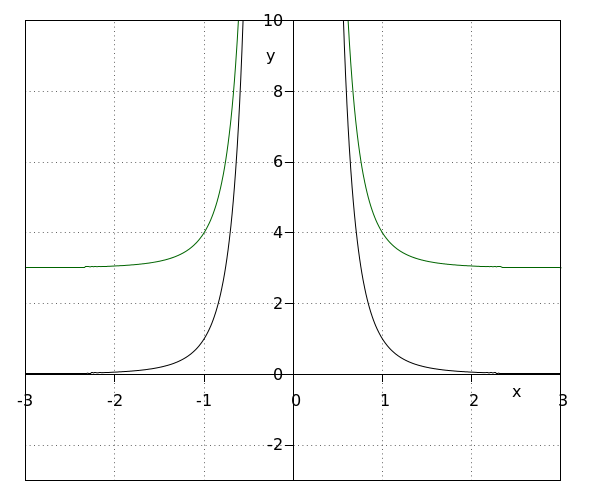
1	We shift the graph of $f(x)=\tfrac{1}{x^n}$ upwards by $\green q$ . The new function is $f(x)=\frac{1}{x^n}+\green q$ Since the new function shifts upwards by $\green q$ , the range becomes equal to $\ivoo{\green q}{\infty}$ when $n$ is even. When $n$ is odd, the range becomes equal to all numbers except $\green q$ . The domain stays the same. Hence, the horizontal asymptote is equal to $y=\green q$ and the vertical asymptote remains equal to $x=0$ .	shifting $f(x)=\frac{1}{x^4}$ upwards by $\green3$ gives $f(x)=\frac{1}{x^4}+\green3$
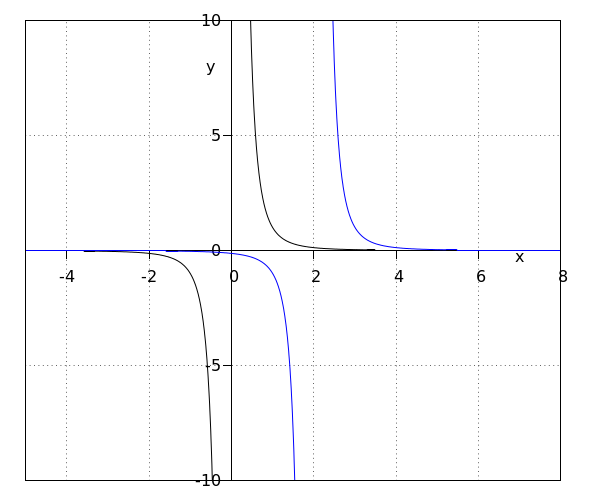
2	We shift the graph of $f(x)=\tfrac{1}{x^n}$ to the right by $\blue p$ . The new function becomes $f(x)=\frac{1}{\left(x-\blue p\right)^n}$ Since the new function is shifted to the right by $\blue p$ , the domain becomes equal to all numbers except $x=\blue p$ . The range remains the same. Hence, the horizontal asymptote remains equal to $y=0$ and the vertical asymptote becomes equal to $x=\blue p$ .	shifting $f(x)=\frac{1}{x^3}$ to the right by $\blue2$ gives $f(x)=\frac{1}{\left(x-\blue2\right)^3}$
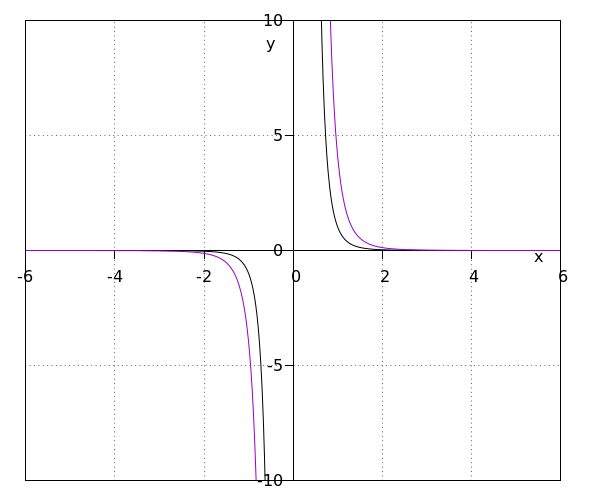
3	We multiply the graph of $f(x)=\tfrac{1}{x^n}$ by $\purple a$ relative to the $x$ -axis. The new function becomes $f(x)=\frac{\purple a}{x^n}$ When multiplying by a positive number, domain, range and asymptotes remain equal to the original function. On the other hand, if we multiply by a negative number, the function reverses. When $n$ is odd, domain, range and asymptotes remain the same. That is not the case when $n$ is even, there the range also reverses to $\ivoo{-\infty}{0}$ . Domain and asymptotes remain the same.	multiplying $f(x)=\frac{1}{x^5}$ by $\purple4$ relative to the $x$ -axis gives $f(x)=\frac{\purple4}{x^5}$

Multiple transformationsWe can also perform multiple transformation after one another.

For example: $f(x)=\frac{\purple3 }{\left(x-\blue4\right)^4}$ is first a shift of $\blue4$ to the right hand side and next a multiplication by $\purple3$ relative to the $x$ -axis, of the power function with negative exponent $f(x)=\frac{1}{x^4}$ .

When shifting to the right by $\blue p$ we also saw a shift in the vertical asymptote. This means that the symmetry axis of an even function shifts to the right by $x=\blue p$ .

A power function with an odd, negative exponent $n$ has a symmetry point at $\rv{0,0}$ . When shifting upwards by $\green q$ , the symmetry point also shifts upwards by $\green q$ . In the same manner when shifting to the right by $\blue p$ the symmetry point also shifts to the right by $\blue p$ to the point $\rv{\blue p, 0}$ .

Take a look at the two graphs below.

The blue (solid) graph has formula:

$y=-{{3}\over{x^4}}$
What is the formula of the green (dashed) graph?

$y=$ $3-{{3}\over{x^4}}$

The point $\rv{1,-3}$ lies on the blue graph, we will investigate where this same point is on the green graph. On the green graph this point lies at $\rv{1,0}$ .
Hence, the green graph is obtained from the blue graph by shifting the blue graph upwards by $3$ .
Hence we add $3$ to the formula for the blue graph $y=-{{3}\over{x^4}}$ . This gives the following formula for the green graph:

$y=3-{{3}\over{x^4}}$

New example